5. Trigonometric Functions and Graphs
Resources for 5. Trigonometric Functions and Graphs
| Site: | ARPDC |
| Course: | ERLCMath 30-1, 2012-2014 - Stephanie MacKay (Click to Enter) |
| Book: | 5. Trigonometric Functions and Graphs |
| Printed by: | Guest user |
| Date: | Thursday, 21 November 2024, 1:04 PM |
5. Trig Functions and Graphs
During the Sept 2013 - Jan 2014 Semester I decided to teach the Sine curve with all of the transformations in two days. After students had a good grasp of the vocabulary and graphing sine curves, I then taught the cosine curve with all of the transformations. This grouping seemed to have worked better with my students as it allowed for repeated use of vocabulary such as amplitude, phase shift, displacement, and period.
5.1 Graphing Sine and Cosine Functions
Class Notes
The McGraw-Hill Ryerson PreCalculus 12 Text is used as the Main Resource.
Assignments in the Powerpoint Lesson Plans refer to pages and questions in the PreCalculus 12 text.
5.1 Graphing Sine and Cosine Functions
5.1 Formative Assessment Amplitude and Period
Digital Resources
Unwrapping the Circle: 5.1 Deroulecercle.ggb
Unit Circle Graphing Sine and Cosine
Pedagogical Shifts: TRANSFORM, Moving from Traditional to Student-Centered
Shifting from Student as Knowledge Recipient to Student as Inquirer and Creator
Shifting from Memorization to Higher-level Thinking
Desmos pre-created interactive graphs for trig functions are available online. Follow this link.
https://www.desmos.com/calculator Click on the bars in the upper left corner to view all pre-created interactive graphs.
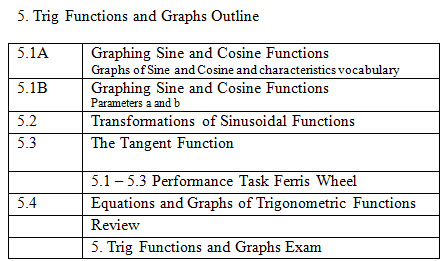
Building a Sine Curve and Making Connections to the Unit Circle.
This is a nice idea to make connections between the unit circle and the graph of the sine function. Students can trace the heights of the curve to build half a period of a sine curve. Possible follow up questions include "What would the other half of the graph look like?" Connections could also be made to characteristics of a sine function graph such as amplitude and period.
Template for Building the sine function graph.
5.2 Transformations of Sinusoidal Functions
Class Notes
The McGraw-Hill Ryerson PreCalculus 12 Text is used as the Main Resource.
Assignments in the Powerpoint Lesson Plans refer to pages and questions in the PreCalculus 12 text.
5.2 Transformations of Sinusoidal Functions
5.2 Formative Assessment Graphing Phase Shift and Displacement
Digital Resources to Enhance Learning and Differentiate Instruction
Pedagogical Shifts: TRANSFORM, Moving from Traditional to Student-Centered
Shifting from Student as Knowledge Recipient to Student as Inquirer and Creator
Shifting from Memoriation to Higher-level Thinking
Shifting from competative to collaborative learning.
Matching Sinusoidal Equations with Graphs and Properties
In this activity, students worked in pairs to match the graph with the function equation and function table. They also had to list the features of the graph including amplitude, period, and principal axis.
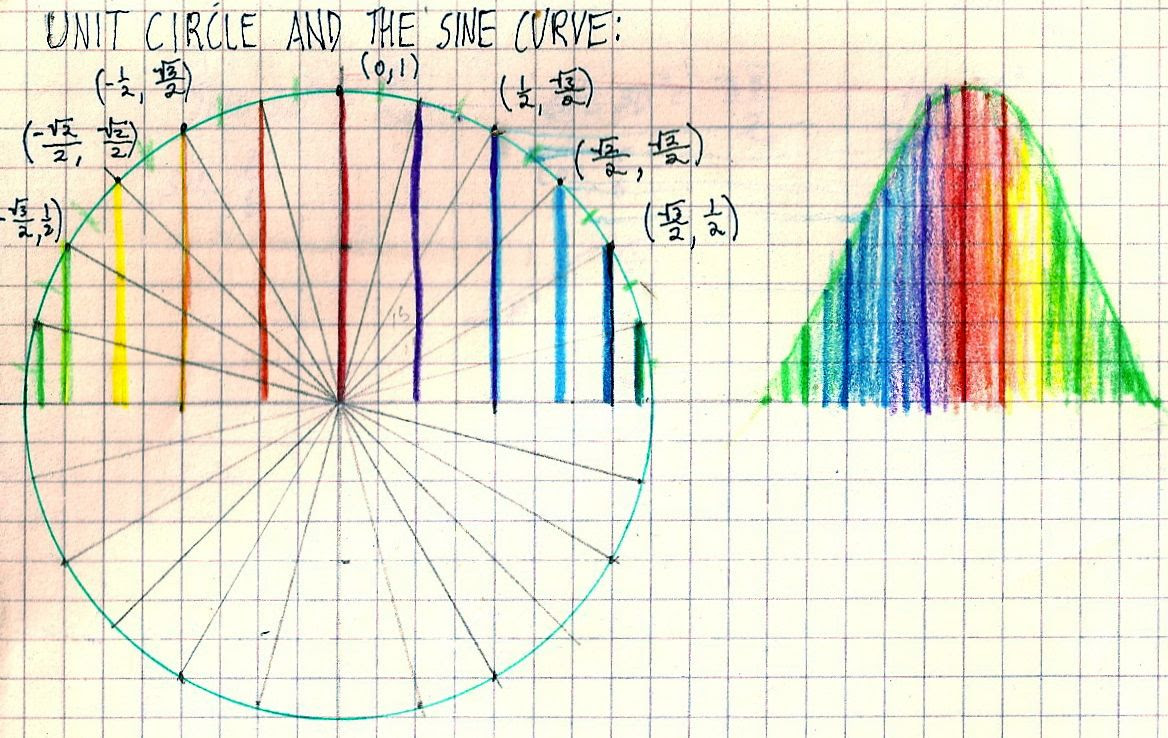
Wave Rider Game
In this game, student must race the timer to write the equation of a sinusoidal function that matches the given graph. Extra point are given if you can collect the token. See who in your class can beat the high score.
http://www.bbc.co.uk/bitesize/higher/games/wave_rider/
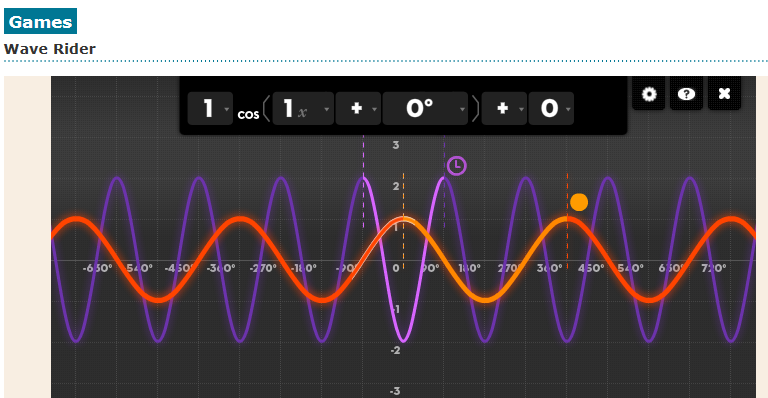
Desmos Sinusoidal Transformations Investigation (Download: SineTransformationsInv.docx)
This investigation assumes that students understand the basic properties of y = sinx and y = cosx and the transformations related to y = a f[b(x - c)] + d. Students will explore how the parameters a, b, c, and d affect sinusoidal functions.
The investigation involves three steps:
1. Use Visualization to predict what characteristics will change when each of the parameters are adjusted.
2. Verify the predictions from step 1 by using Desmos and sliders for each parameter.
3. Explore in more detail the relationship between the parameters and the characteristics of the graph.
Investigation - Transformations Connections (Download: TransformationsConnections.docx)
This activity asks students to review a transformation on a function they are already familiar with and then apply the same type of transformation to a sinusoidal function.
This is an example of making Connections between mathematical concepts. Visit the Connections Process Page to explore more ways to incorporate Connections in your teaching.
5.3 Tangent Function
Class Notes
The McGraw-Hill Ryerson PreCalculus 12 Text is used as the Main Resource.
Assignments in the Powerpoint Lesson Plans refer to pages and questions in the PreCalculus 12 text.
5.3 Graphing Tangent Functions Formative Assessment
Digital Resources
Interactive Unit Circle Applet
http://www.mathsisfun.com/algebra/trig-interactive-unit-circle.html
5.4 Equations and Graphs of Trigonometric Functions
Class Notes
The McGraw-Hill Ryerson PreCalculus 12 Text is used as the Main Resource.
Assignments in the Powerpoint Lesson Plans refer to pages and questions in the PreCalculus 12 text.
5.4 Equations and Graphs of Trigonometric Functions
5. Graphing Trig Functions Performance
5.4 Formative Assessment Graphing Trig Functions
Digital Resources
Pedagogical Shifts: TRANSFORM, Moving from Traditional to Student-Centered
Shifting from Content-based to Competencies-based
Shifting from Student as Knowledge Recipient to Student as Inquirer and Creator
Shifting from Print-based to Multimodal
I used this short video to allow students to create the equation of the function of the height of a car on a ferris wheel to time. Students had to ask questions and relate the features of the ferris wheel to the values on the graph and the parameters of the sinusoidal function equation. They had to discover how to change the horizontal axis from radians to time and the effect that would have in the function equation. Student were able to discover that the best function equation (in their opinion) was a negative cosine function.
Ferris Wheel Matching Activity
In this activity, students must solve problems involving ferris wheels. In the first problem, students are given dimensions and features of a ferris wheel and need to graph height of a car over time. The middle portion of the activity, students must match graphs, equations, and features of different ferris wheels. Students then finish the activity with problem similar to the first problem.
