Quadratic Functions - Engaging Resources
![]()
RF SO1 - Quadratic Functions Videos
These videos may be useful in various ways. The "Giant Slide" may be used as an interesting introduction to parabolas and begin a discussion on whether we should believe everything we see on YouTube. "Parabolas in the Real World" provides a number of nice examples and the "Review Video" quickly touches upon many aspects of Quadratic Functions.
7 Processes focus: Technology. Visit the Technology Process page for more ideas on how to incorporate Technology in your teaching.
![]()
RF SO1 - Characteristics from Functions Chart
(Download: Blank Chart.docx, Completed Chart.docx)
Students love this chart as a study tool and a way to see the big picture. It was assisted in determining the various strengths of the different forms of the quadratic function. Lessons may simply consist of completing each section of the chart and providing students with some practice on that skill.
7 Processes focus: Connections. Visit the Connections Process page for more ideas on how to incorporate Connections in your teaching.
![]()
RF SO1 - JigSaw Activity: Forms of Quadratic Functions
(Download: JigSaw Equation Forms.docx)
Follow the link to learn more about JigSaw Activities. This JigSaw is fun in that when the groups get back together they will all realize that they graphed the same function even though the equations did not look the same. This reinforces the idea that one quadratic function may be in various forms.
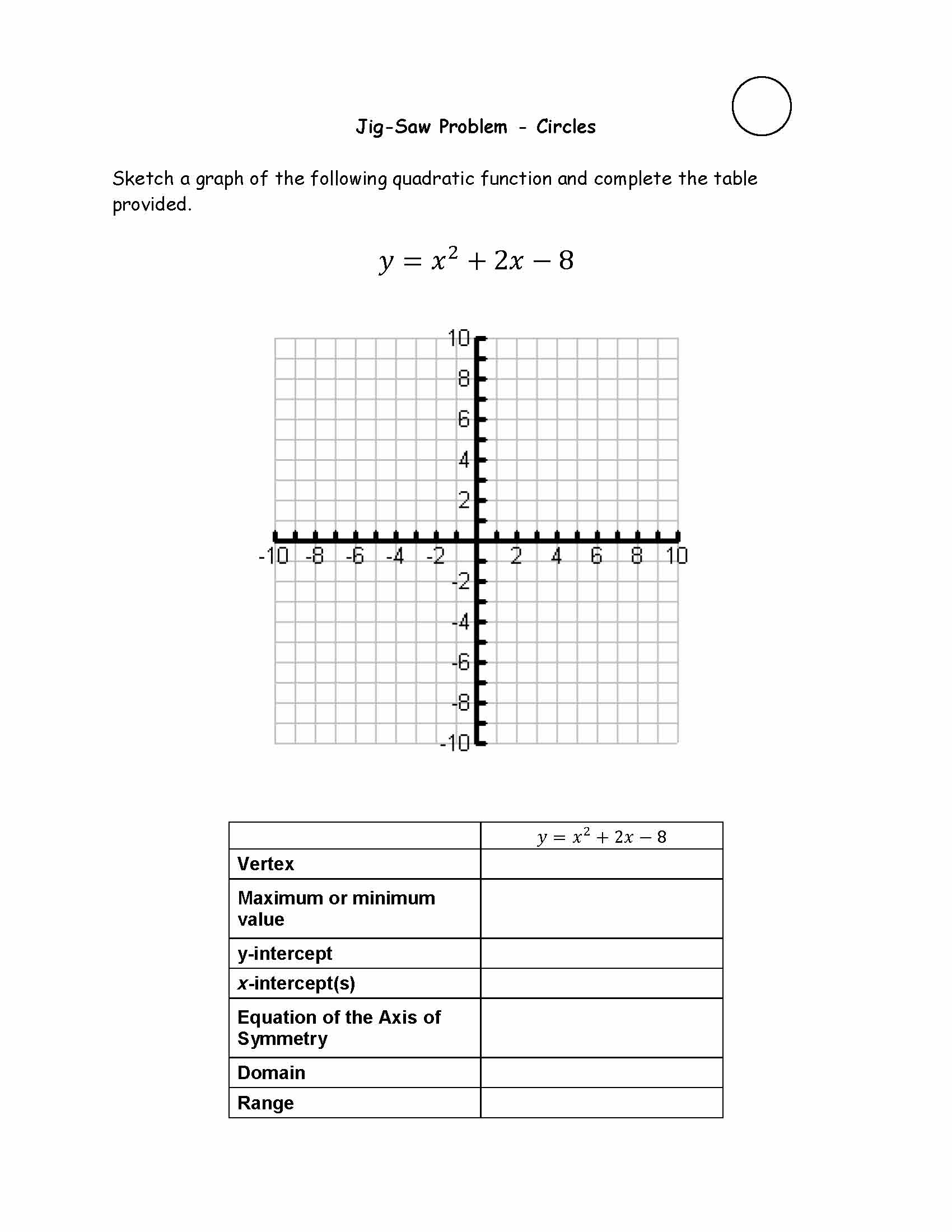
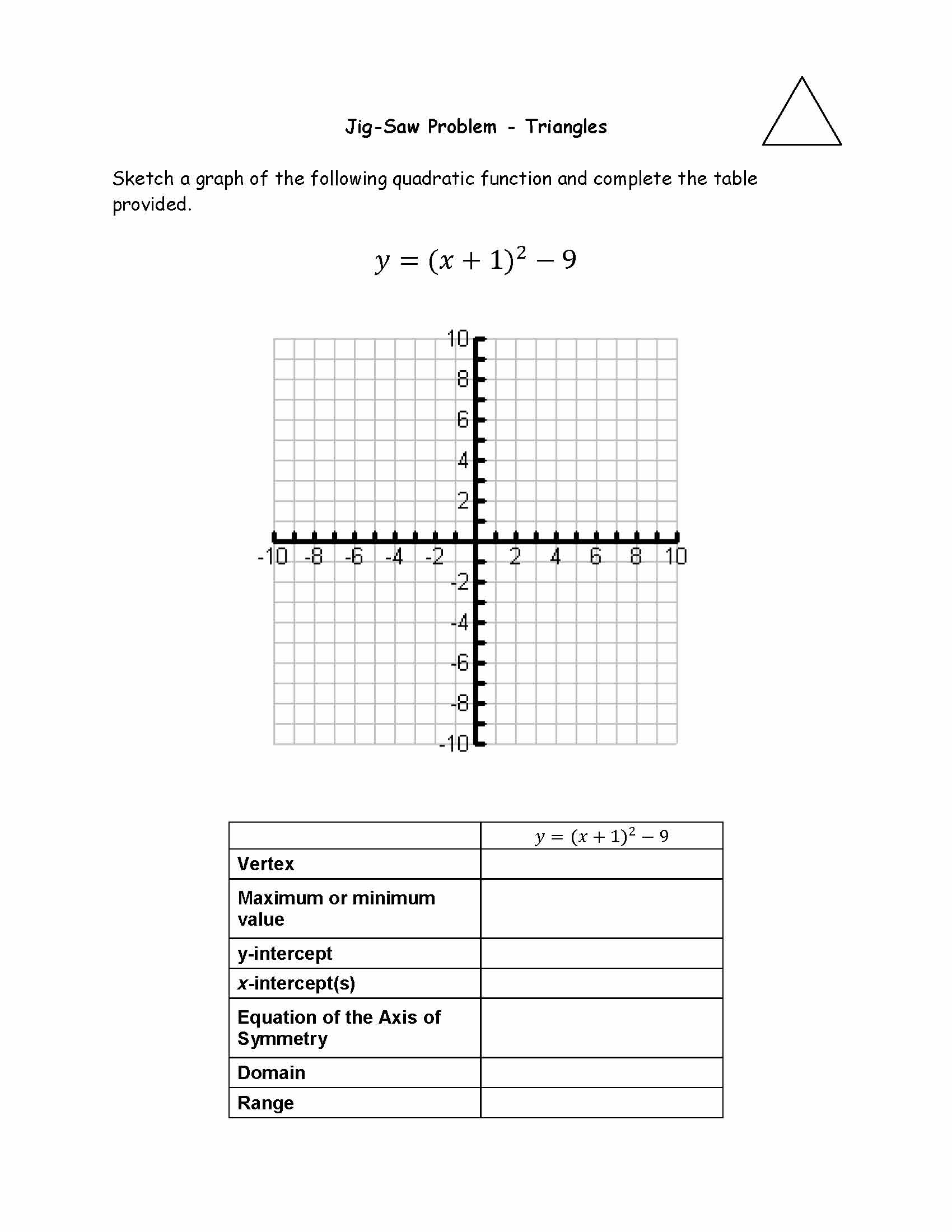
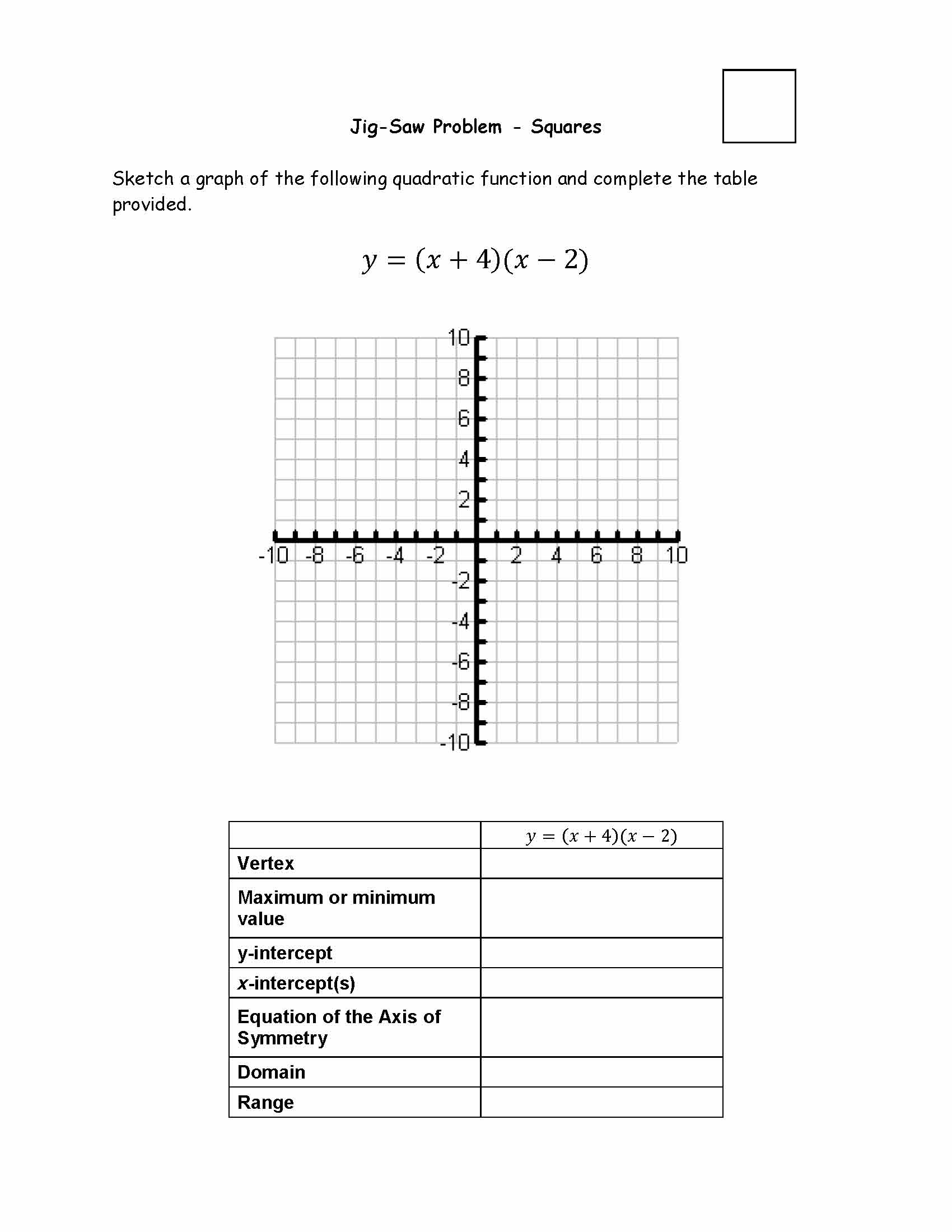
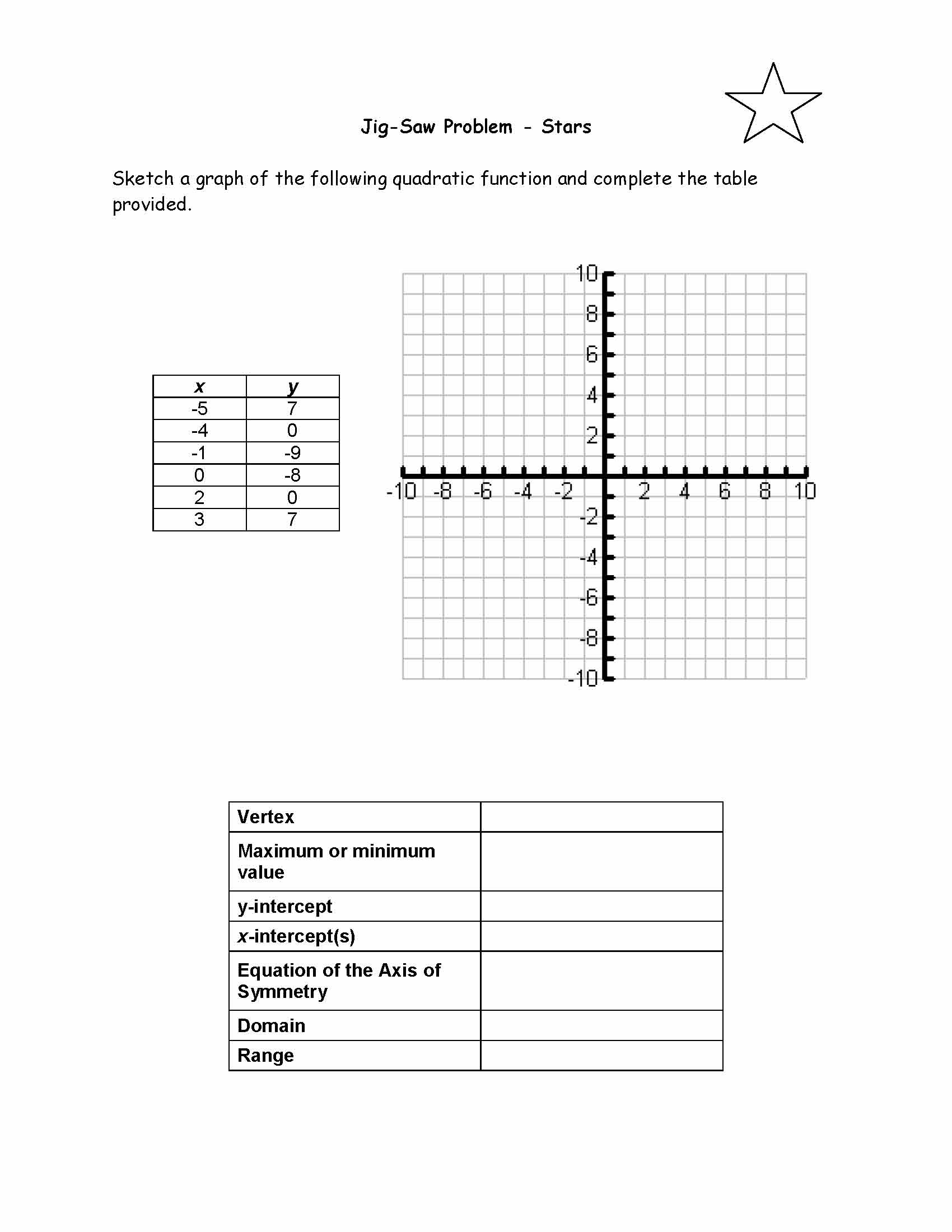
7 Processes focus: Communication. Visit the Communication Process page for more ideas on how to incorporate Communication in your teaching.
![]()
RF SO1 - Modelling Quadratics
(Link: TeachMathematics.Net)
Quadratic graphs are everywhere you look. They can describe the paths of rockets, balls and jets of water. Because of their symmetry you will see parabolas in bridges, buildings, sand dunes... Understanding their equations is hence hugely important in the fields of engineering and science. This activity uses dynamic geometry software to help students understand the graph of quadratics in the form y = a(x - b)² + c.
7 Processes focus: Connections. Visit the Connections Process page for more ideas on how to incorporate Connections in your teaching.
7 Processes focus: Technology. Visit the Technology Process page for more ideas on how to incorporate Technology in your teaching.