Rational Expressions Unit Plan
Counting Methods
| Site: | ARPDC |
| Course: | ERLC Math 30-2, 2012-2014 - Candace Ketsa (Click to Enter) |
| Book: | Rational Expressions Unit Plan |
| Printed by: | Guest user |
| Date: | Thursday, 16 October 2025, 5:20 PM |
Table of contents
Introduction to Rational Expressions and Equations
General Outcome: Develop algebraic and graphical reasoning through the study of relations.
SO 1. Determine equivalent forms of rational expressions (limited to numerators and denominators that are monomials and binomials).[C, ME, R]
1.2 Explain why a given value is non-permissible for a given rational expression.
1.3 Determine the non-permissible values for a rational expression.
1.4 Determine a rational expression that is equivalent to a given rational expression by multiplying the numerator and denominator by the same factor (limited to a monomial or a binomial), and state the non-permissible values of the equivalent rational expression.
1.5 Simplify a rational expression.
1.6 Explain why the non-permissible values of a given rational expression and its simplified form are the same.
1.7 Identify and correct errors in a given simplification of a rational expression, and explain the reasoning.
SO2. Perform operations on rational expressions (limited to numerators and denominators that are monomials and binomials). [CN, ME, R]
2.1 Compare the strategies for performing a given operation on rational expressions to the
strategies for performing the same operation on rational numbers.
2.2 Determine the non-permissible values when performing operations on rational expressions.
2.3 Determine, in simplified form, the sum or difference of rational expressions that have the same denominator.
2.4 Determine, in simplified form, the sum or difference of two rational expressions that have different denominators.
2.5 Determine, in simplified form, the product or quotient of two rational expressions.
SO3. Solve problems that involve rational equations (limited to numerators and denominators that are monomials and binomials). [C, CN, PS, R]
3.1 Determine the non-permissible values for the variable in a rational equation.
3.2 Determine, algebraically, the solution to a rational equation, and explain the strategy used to solve the equation.
3.3 Explain why a value obtained in solving a rational equation may not be a solution of the equation.
3.4 Solve a contextual problem that involves a rational equation.
Mathematical Processes
- Communications [C]
- Connections [CN] Students are expected to make connections among mathematical ideas, other concepts in mathematics, everyday experiences and other disciplines
- Problem Solving [PS] Students are expected to develop and apply new mathematical knowledge through problem solving
- Reasoning [R] Students are expected to develop mathematical reasoning
- Mental Estimation [ME] Students are expected to demonstrate fluency with mental mathematics and estimation.
Review
Students will need a good foundation of factoring binomials.
________________________________________________________________
Lesson Links:
- Click here for a Notebook version of ERLC Lesson Link. Please use this lesson as a framework for your own teaching environment.
- Click here for a pdf version of the same ERLC Lesson Link.
________________________________________________________________
Additional Resources:
Factoring Flow Chart -- Bauer
Factoring Review Notes (Including Trinomials) -- Bauer
Fraction Review -- Dutchak (Use day 1 as a warm-up and a great reference sheet to come back to throughout the ‘Rational Expression’ unit)
Assessment for Learning
Worksheets:
Factoring Binomials AFLs -- Modified from Bauer Review Sheet below
Factoring Review Sheet (Includes Trinomials) -- Bauer
Equivalent Rational Expressions
Achievement Indicators:
1.1 Compare the strategies for writing equivalent forms of rational expressions to writing equivalent forms of rational numbers.
1.2 Explain why a given value is non-permissible for a given rational expression.
1.3 Determine the non-permissible values for a rational expression.
1.4 Determine a rational expression that is equivalent to a given rational expression by multiplying the numerator and denominator by the same factor (limited to a monomial or a binomial), and state the non-permissible values of the equivalent rational expression.
______________________________________________________________________
Lesson Links:
- Click here for a Notebook version of ERLC Lesson Link. Please use this lesson as a framework for your own teaching environment.
- Click here for a pdf version of the same ERLC Lesson Link.
______________________________________________________________________
Additional Resources:
Absolute Value Workbook: Lessons 1 and 2 on Pages 417 - 432
Discovery Based Learning
Absolute Value Workbook -- Investigating Non-Permissible Values Page 418/419 [CN]
Simplifying Rational Expressions
Achievement Indicators:
1.2 Explain why a given value is non-permissible for a given rational expression.
1.3 Determine the non-permissible values for a rational expression.
1.4 Determine a rational expression that is equivalent to a given rational expression by multiplying the numerator and denominator by the same factor (limited to a monomial or a binomial), and state the non-permissible values of the equivalent rational expression.
1.5 Simplify a rational expression.
1.6 Explain why the non-permissible values of a given rational expression and its simplified form are the same.
1.7 Identify and correct errors in a given simplification of a rational expression, and explain the reasoning.
______________________________________________________________________
Lesson Links:
- Click here for ERLC Lesson Link. Please use this lesson as a framework for your own teaching environment
- Click here for a pdf version of the same ERLC Lesson Link.
______________________________________________________________________
Additional Resources:
Notes on Simplifying Rational Expressions
Notes on Simplifying Rational Expression -- Barry
Assessment for Learning
Worksheets:
Simplying Rational Expressions Assessment for Learning Questions -- Barry
Peer Assessments:
How Simple Is Your Rational Expression? Checklist
Assign the worksheet and the next day have them work in partners for peer assessment.
Partner Game -- Learn Alberta
With a partner, complete the following activity. Each of you will answer a set of math questions. One player will answer the questions in the Player A column. The other person will answer the questions in the Player B column. When you’re both done, compare your answers. Your answers should match in each row. If the answers do not match, then work together to figure out where you went wrong. Remember to state the non-permissible values!
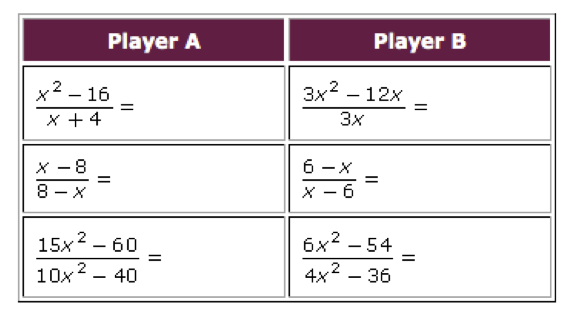
Journal Prompts [C]
- What are some key differences between simplifying rational numbers and simplifying rational expressions?
- Why is it necessary to include the non-permissible value(s) with the simplified form even though this form may not have a denominator?
- Based on your answer to question 2, when should you identify non-permissible values?
Multiplying and Dividing Rational Expressions
Achievement Indicators:
2.1 Compare the strategies for performing a given operation on rational expressions to the
strategies for performing the same operation on rational numbers.
2.2 Determine the non-permissible values when performing operations on rational expressions.
2.5 Determine, in simplified form, the product or quotient of two rational expressions.
______________________________________________________________________
Lesson Links:
- Click here for ERLC Lesson Link. Please use this lesson as a framework for your own teaching environment.
- Click here for a pdf version of the same ERLC Lesson Link.
_________________________________________________________
Discovery Based Learning
Discovery - Multipication of Rational Expressions -- Ketsa
This activity conceptualizes that the rule of multiplication of fractions holds true for mutliplying rational expressions.
Discovery - Division of Rational Expressions -- Ketsa
This activity conceptualizes that the rule of division of fractions holds true for dividing rational expressions.
Assessment for Learning
This is a fun way to assess the concept of multiplying rational expressions (monomials). This could definatley be adapted to add in rational expressions that are binomials as well.
Journal Prompts
- How are the processes of multiplying
 and
and  similar? How are they different?
similar? How are they different?
- Is it necessary to expand an expression such as
 before multiplying? Explain. Why is factoring a useful step in simplifying an expression?
before multiplying? Explain. Why is factoring a useful step in simplifying an expression?
Adding and Subtracting Rational Expressions
Achievement Indicators:
2.1 Compare the strategies for performing a given operation on rational expressions to the strategies for performing the same operation on rational numbers.
2.2 Determine the non-permissible values when performing operations on rational expressions.
2.3 Determine, in simplified form, the sum or difference of rational expressions that have the same denominator.
2.4 Determine, in simplified form, the sum or difference of two rational expressions that have different denominators.
______________________________________________________________________
Lesson Links:
- Click here for ERLC Lesson Link. Please use this lesson as a framework for your own teaching environment.
- Click here for a pdf version of the same ERLC Lesson Link.
______________________________________________________________________
Additional Resources:
Notes on Adding and Subtracting Rational Expressions -- Barry
Solving Rational Equations
Achievement Indicators:
3.1 Determine the non-permissible values for the variable in a rational equation.
3.2 Determine, algebraically, the solution to a rational equation, and explain the strategy used to solve the equation.
3.3 Explain why a value obtained in solving a rational equation may not be a solution of the equation.
3.4 Solve a contextual problem that involves a rational equation.
______________________________________________________________________
Lesson Links:
- Click here for ERLC Lesson Link. Please use this lesson as a framework for your own teaching environment.
- Click here for a pdf version of the same ERLC Lesson Link.
______________________________________________________________________
Student Exemplars
Please feel free to share any student's work by emailing me at cketsa@gsacrd.ab.ca
Summative Assessments
Click here to assess the summative assessment area. You will need an enrolment key to access this portion of the site. Please email cketsa@gsacrd.ab.ca for access.
Below is a list of what is available for the Unit.
_______________________________________________________________________________________________________________________
Assignments
1. Unit Assignment with Answer Key -- Ketsa
2. Rational Expressions Worksheet -- Fowler
Quizzes
1. Factoring Quiz -- Bauer
2. Quizzy 1 - Simplifying -- Andersen
3. Quizzy 2 - Adding and Subtracting -- Andersen
4. Quizzy 3 - Mulitplying and Dividing Rational Expressions - Andersen
5. Quizzy 4 - Solving Rational Equations -- Andersen
6. Rational Expressions - UNIT QUIZZY -- Andersen
7. RE Quiz with Answer Key -- Fowler
Exams
1. Probability Unit Exam with Blueprint and Answer Key -- Ketsa
2. Summative Test 1 - Rational Expressions, Polynomials -- Andersen
3. Rational Expressions Unit Exam with Answer Key -- Kalemba
Project Based Assessment